In early chapters, we introduced the concept of ticks, which discretize the price curve. A tick is a price defined by the formula $p(i)=1.0001^i$, where $i$ is named tick index.
Tick indexes are integers within the range $[-887272,887272]$, resulting in 1,774,545 ticks along the price curve, from $p(-887272)$ to $p(887272)$.
Ticks are points on the curve where liquidity may change. For example, a liquidity provider can add liquidity between two ticks but cannot do so between two arbitrary points that are not ticks.
What we will see in this chapter is that not all of these 1,774,545 ticks can be used in a pool, and the specific ticks that can be used depend on a choice made at the time of creating the pool.
Each pool defines a value called tick spacing, which determines the distance between two consecutive allowed ticks.
For instance, if the tick spacing of the pool is set to 10, only tick indexes that are multiples of 10 are usable, such as -20, -10, 0, 10, 20, etc. If the tick spacing is set to 60, only multiples of 60 are allowed, such as -120, -60, 0, 60, 120, etc, as illustrated in the figure below. In both scenarios, a tick such as 55 cannot be used as a boundary for providing liquidity.
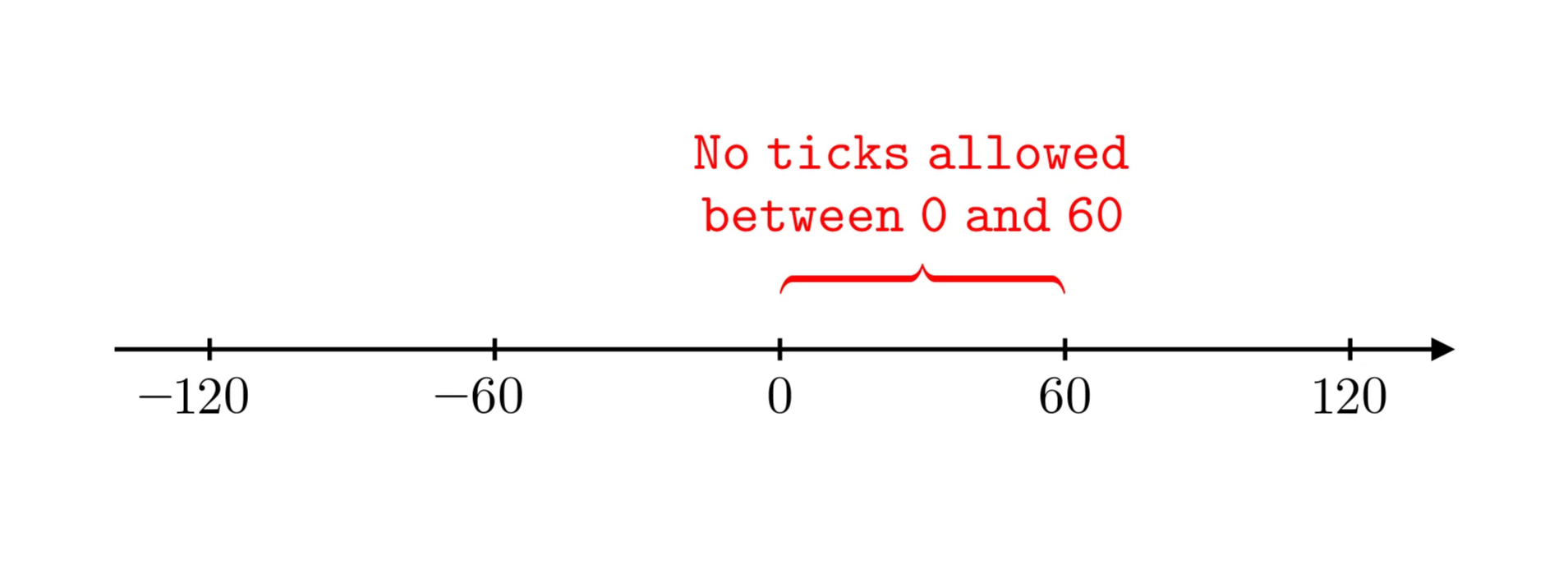
The variable that defines tick spacing in a pool is named tickSpacing and is set at the time of pool creation. In fact, tickSpacing is linked to the pool fee, with each fee tier determining a corresponding tick spacing.
Uniswap V3 supports different tick spacings to account for the varying volatility of asset pairs. Crossing a tick in a swap incurs a gas cost, so ticks should be crossed as infrequently as possible during a typical trade.
More volatile pairs benefit from wider tick spacing to reduce excessive tick crossings. However, if the spacing is too wide, liquidity providers cannot precisely position their liquidity around the price regions where they expect the pair’s market value to be.
See the animation below, where we present two cases. In the first case, involving a highly volatile pair, the price can vary significantly. So, if we want to try to limit swaps to crossing only two allowed ticks, the distance between allowed ticks must be large. In the second case, involving a more stable pair, the tick spacing can be smaller.
Also, consider the risk of impermanent loss for liquidity providers. Highly volatile assets tend to cause higher impermanent loss, while more stable assets tend to cause less impermanent loss. For instance, a stablecoin pair has virtually no risk of impermanent loss, while a memecoin pair carries an extremely high risk.
Thus, LPs will demand higher fees to compensate for impermanent loss when providing liquidity for highly volatile assets. Similarly, traders can tolerate higher fees on volatile assets since the potential returns from trading them are much greater.
This indicates that the relationship between pair volatility, tick spacing, and fees should be as follows: for volatile pairs with a high risk of impermanent loss, both tick spacing and fees should be higher, while for more stable pairs with a low risk of impermanent loss, both can be lower.
It is up to the user to decide which pairs are more stable or volatile. What the protocol defines is the relationship between tick spacing and fee tier.
The Uniswap V3 factory contract provides some default values, but Uniswap governance can add additional tick-spacing-fee pairs. This is the subject of the next section.
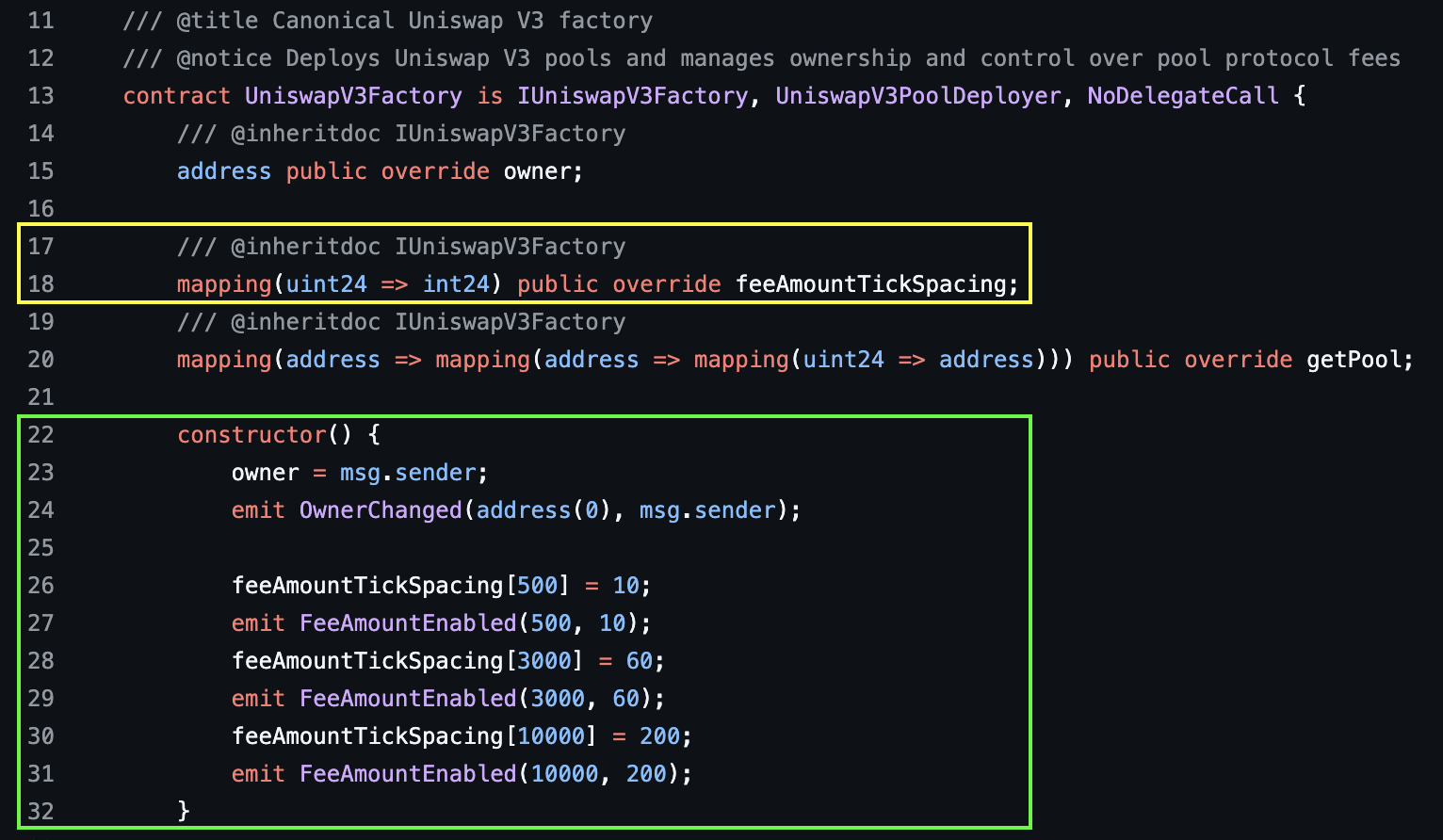
The relationship between fee and tick spacing is contained in the mapping feeAmountTickSpacing (yellow box in the figure below) in the UniswapV3Factory.sol contract. The factory contract is responsible for creating new pools.
The initial relationship between fee and tick spacing was defined during the deployment of the Factory contract, as shown in the constructor in the image below (green box).
The current relationship between fee and tick spacing is shown in the following table. There is no strict “mathematical” relationship between the fee and tick spacing. It’s up to protocol governance to decide the optimal fee for a given tick spacing.
| fee tier | fee tier (basis points) | tick spacing |
|---|---|---|
| 0.01% | 1 | 1 |
| 0.05% | 5 | 10 |
| 0.3% | 30 | 60 |
| 1% | 100 | 200 |
As we can see, a fee of 0.01% corresponds to a tick spacing of 1, while a fee of 0.3% corresponds to a tick spacing of 60.
Fees are measured in basis points. One basis point is 1/100th of 1 percent, or 0.01%. For example, 5 basis points is 5 * 0.01%, or 0.05%.
The mapping from fee to tick spacing can be updated using the function enableFeeAmount, which can only be called by governance.
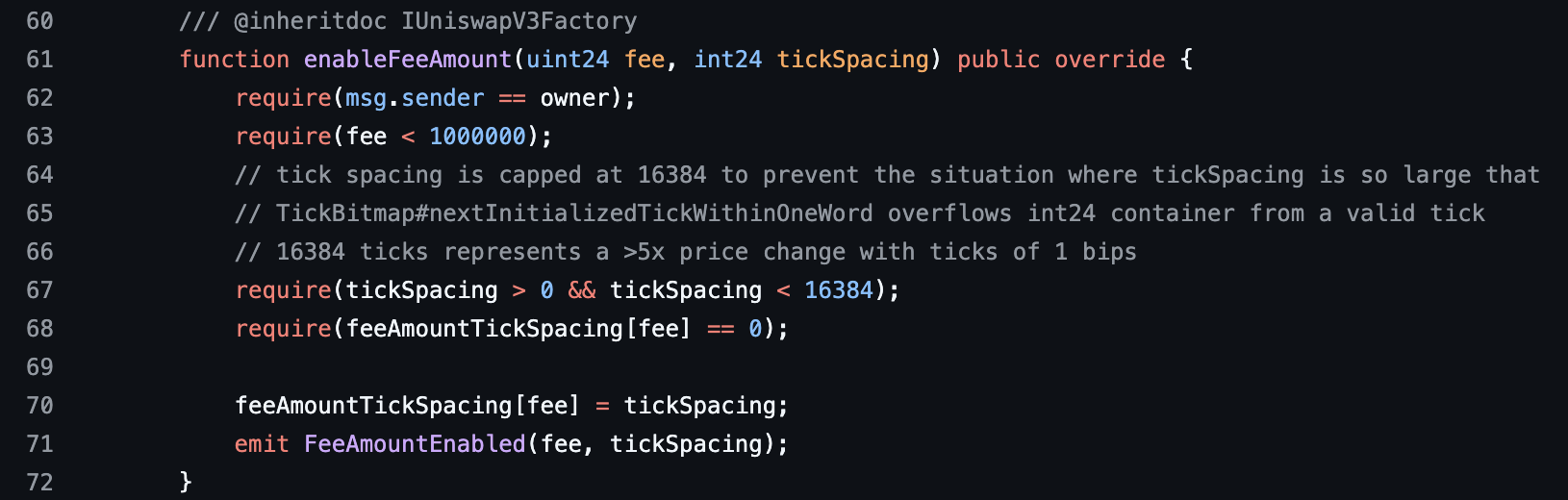
The 1 basis point tier (the 0.01% fee) is not in the constructor, but Uniswap governance added the 1 basis point fee tier on March 5, 2022. You can see the transaction on the tally dashboard.
The available fee tiers can vary on different chains. For example, on the L2 Base, fee tiers for 2, 3, and 4 basis points are available. This addition was executed by governance on September 16, 2024.
To create a new pool, a user must pass in the addresses of both tokens and the desired fee tier. The tick spacing is then calculated from the feeAmountTickSpacing mapping.
A pool is uniquely defined by these three parameters. Thus, it is possible to create pools with the same token pair but different fee tiers. In this case, the market determines which of these pools becomes the ‘popular’ one.
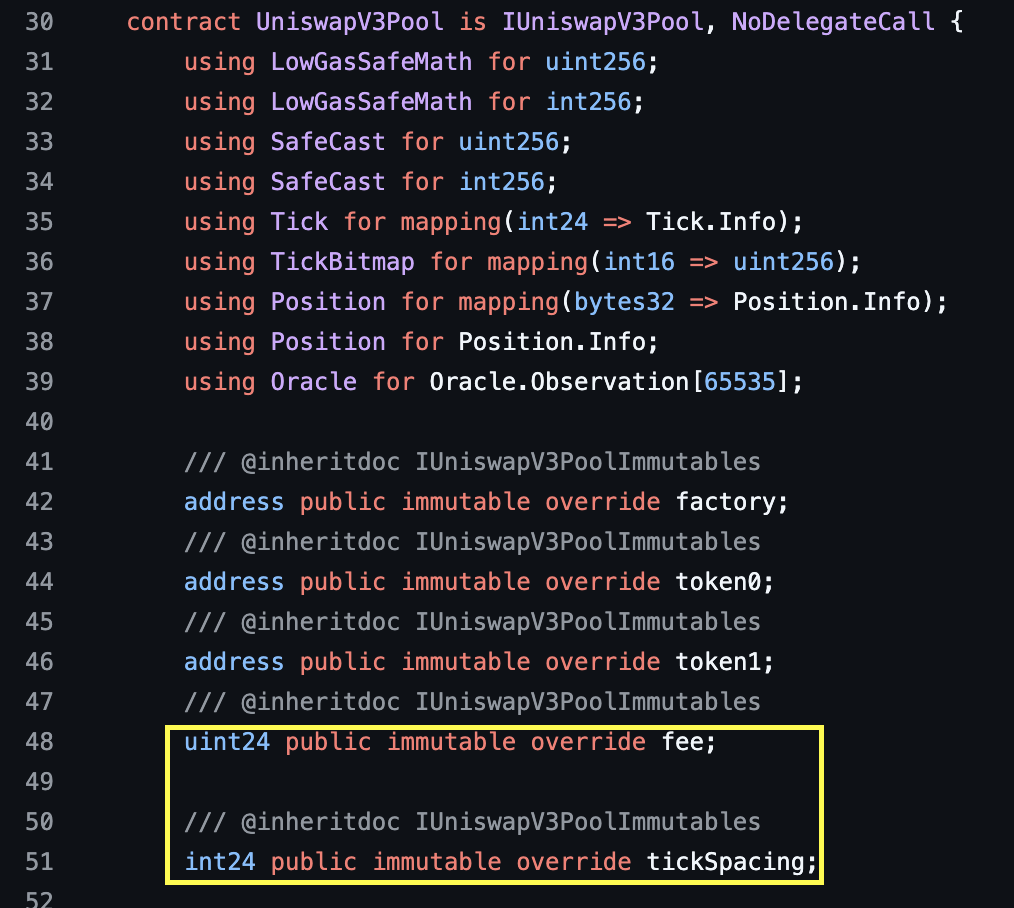
When the pool is deployed by the factory contract, it is passed both the fee and the tickSpacing and these are set as public immutable variables.
Note that there are no restrictions on who can call createPool — it is permissionless as long as the pool for that token pair and fee tier hasn’t been created yet.
Both of these immutable variables (fee and tickSpacing) are public in UniswapV3Pool.sol. In fact, all of the arguments passed to the pool’s constructor are stored in public immutable variables:
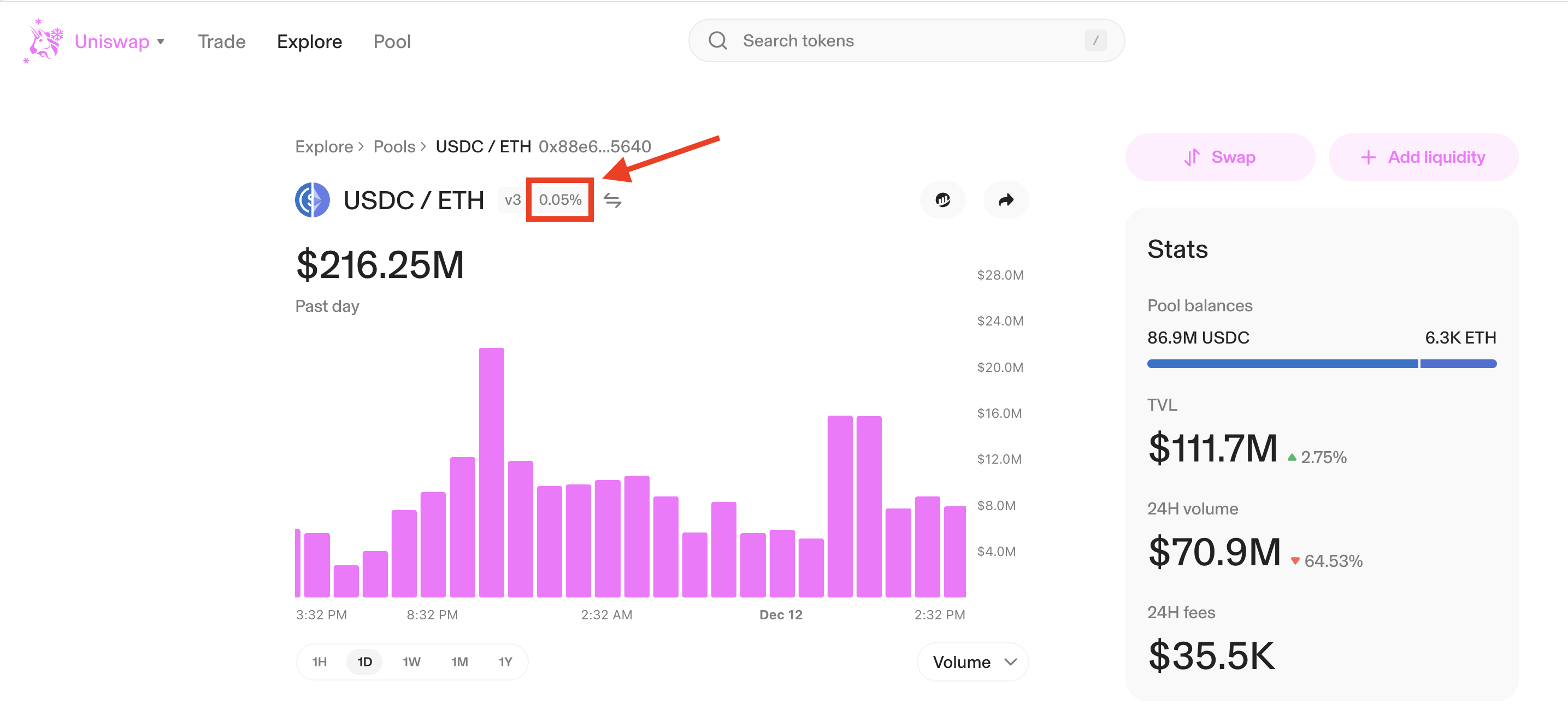
Let’s use the USDC/ETH Pool as a running example. We can see the fee is 5 basis points and it has a tick spacing of 10:
This is how the frontend for that pool knows the fee tier is 0.05%.
Look through the Uniswap V3 Pools.
ZK Proof of Selection Sort Most computations of interest are generally “stateful” — that is, they need to go through a series of steps to produce the final result. Sometimes, we do not need to show we executed the computation but only show the result. For example, if A is a list, we can prove […]
How a ZKVM Works A Zero-Knowledge Virtual Machine (ZKVM) is a virtual machine that can create a ZK-proof that verifies it executed a set of machine instructions correctly. This allows us to take a program (a set of opcodes), a virtual machine specification (how the virtual machine behaves, what opcodes it uses, etc), and prove […]
The Permutation Argument A permutation argument is a proof that two lists hold the same elements, but possibly in a different order. For example, [2,3,1] is a permutation of [1,2,3] and vice-versa. The permutation argument is useful for proving one list is a sorted version of another. That is, if list B has the same […]
ZK Friendly Hash Functions ZK-friendly hash functions are hash functions that require much fewer constraints to prove and verify than traditional cryptographic hash functions. Hash functions such as SHA256 or keccak256 make heavy use of bitwise operators such as XOR or bit rotation. Proving the correct execution of XOR or bit rotation requires representing the […]
As a RareSkills researcher, you will be contributing to the technical content we post on our website.
We’re looking for someone to design and implement security measures and defense-in-depth controls to prevent and limit vulnerabilities.
We’re looking for a Senior Full-Stack Engineer to play a foundational role in working across the entire offchain stack of products.
We are seeking a talented Rust Developer to build a robust, scalable blockchain indexers and analytic backend.